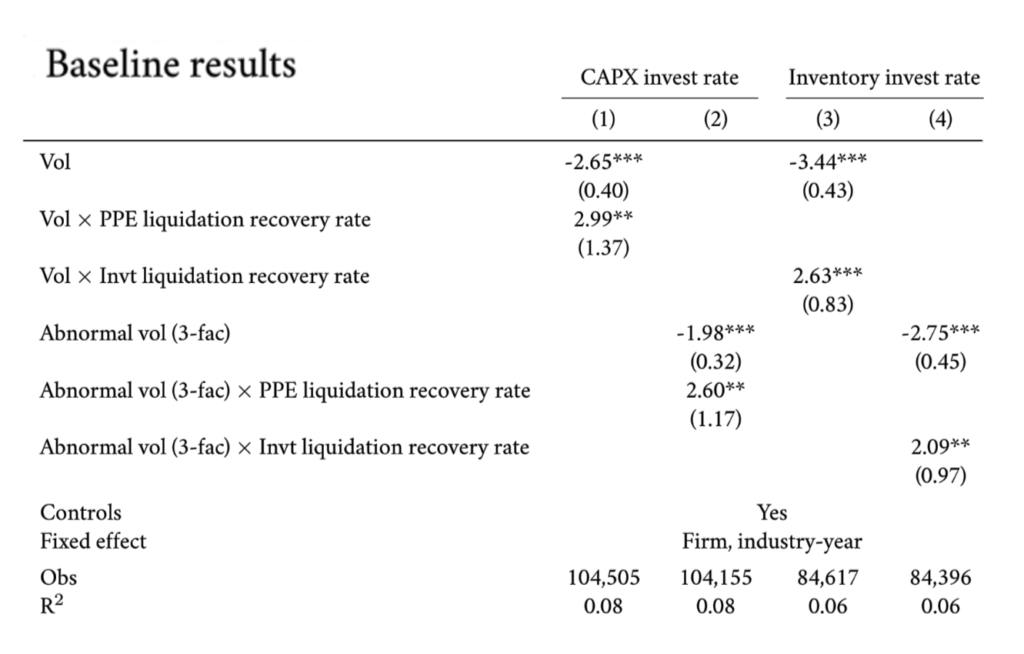
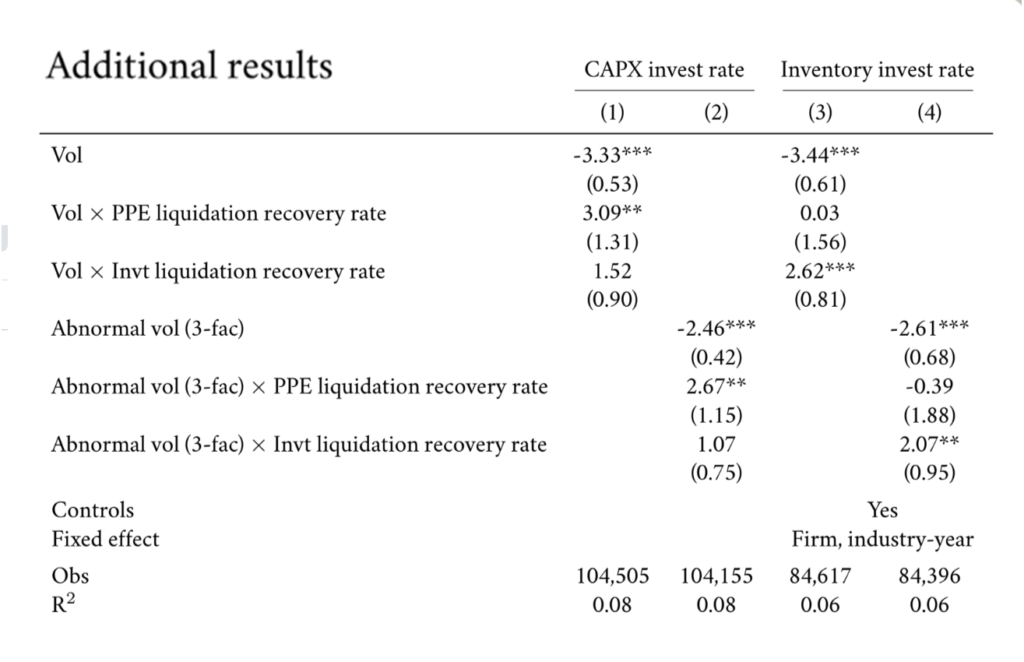
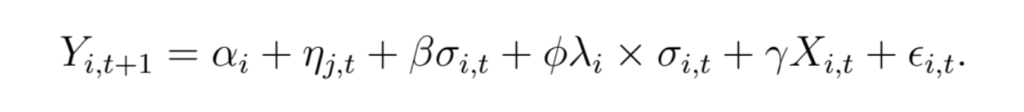For the uncertainty measure σi,t, we use both the daily volatility of a firm’s stock returns over the previous year and the volatility of abnormal returns (based on the Fama-French three factor model) a la Gilchrist, Sim, and Zakrajšek (2014). The liquidation recovery rate is denoted by λi, which is matched to Compustat firms based on their industries. The outcome Yi,t+1 is the investment rate in year t+1 to allow for lags in investment implementation (Lamont, 2000). This specification also alleviates concerns about a reverse impact of investment behavior on stock return volatility. The control variables X include Q, book leverage, cash holdings, EBITDA, size (log book assets), and ratings at the end of year t, as well as the level of stock returns in year t and its interaction with λ. We include firm fixed effects (αi) and industry-year fixed effects (ɳ j,t), and double-cluster standard errors by firm and time. We use a longer sample of 1980 to 2016 to allow for more variation in uncertainty over time.
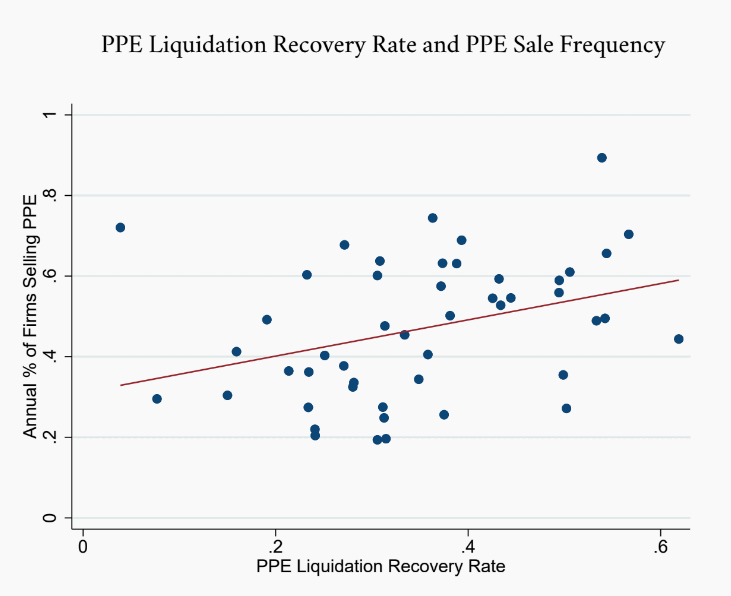
We start with capital expenditures (i.e., investment in PPE) as the outcome variable, normalized by lagged net PPE. In this case, we use the PPE liquidation recovery rate for λ. We find that higher uncertainty is associated with significant decreases in capital expenditures when the PPE liquidation recovery rate is low, but not when the PPE liquidation recovery rate is high. Indeed, when the PPE liquidation recovery rate is zero, the coefficient on volatility (β) is significantly negative; when the PPE liquidation recovery rate is one, the coefficient on volatility (β+Φ) becomes roughly zero. This result matches closely with theoretical predictions and indicates that asset specificity is key to the negative impact of uncertainty on firm investment.